The Linda Problem: When Our Brain Tricks Us Into The Impossible
Have you Ever Been 100% Convinced of Something that Was Mathematically Impossible?
This is The Curious Mind, by Álvaro Muñiz: a newsletter where you will learn about technical topics in an easy way, from decision-making to personal finance.
Our brains are naturally wired to favor compelling stories over mathematical truth.
This cognitive blind spot leads even the most intelligent people to commit what psychologists call the "conjunction fallacy," a fundamental error in probabilistic reasoning that affects up to 90% of us. Psychologists Daniel Kahneman and Amos Tversky demonstrated this phenomenon through their famous "Linda problem," revealing how our intuitive thinking processes consistently misleads when evaluating probabilities. The implications extend far beyond academic puzzles, affecting everything from how we evaluate news stories to how we make critical financial and medical decisions.
Today we explore this mental trap to help us make better judgments under uncertainty.
The Linda Problem
Let me introduce you to a puzzle that confuses most people, including well-educated people in statistics and probability:1
Linda is thirty-one years old, single, outspoken, and very bright. She majored in philosophy. As a student, she was deeply concerned with issues of discrimination and social justice, and also participated in antinuclear demonstrations.
What is more probable?
Linda is a bank teller
Linda is a bank teller and is active in the feminist movement
The Surprising Truth
If you chose option 2, you've just fallen for one of the most fascinating cognitive traps in human reasoning.
You are not alone. When psychologists Daniel Kahneman and Amos Tversky presented this question to undergraduate students across several US universities, a staggering 85-90% selected option 2.
But here's the bombshell: Option 2 cannot possibly be more likely than Option 1.
Why Our Brains Get It Wrong
When we read Linda's description, our mind creates a story. We can almost see her—the passionate philosophy student fighting for social justice. Our brain searches for the most fitting conclusion to this character sketch, and "bank teller who's also a feminist" simply feels right.
It creates a coherent narrative. It resonates with the character we've imagined.
But this is where our intuition misleads us.
The Mathematical Reality (Simplified)
Here is the essential problem with saying Option 2 is more likely:
Every bank teller who is active in the feminist movement is also a bank teller.
Think about it this way:
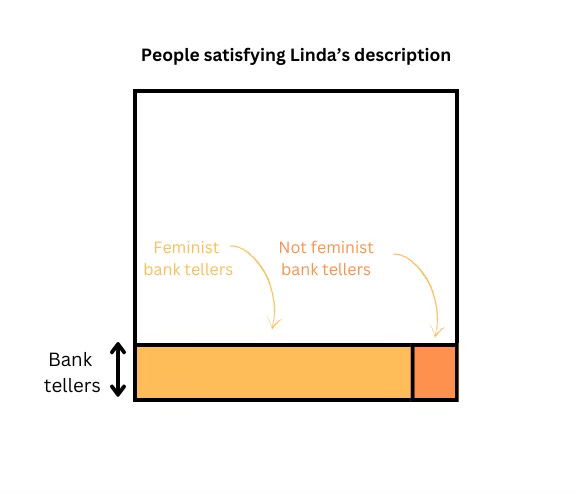
Imagine 100 people who match Linda's description:
Let's say 10 of them are bank tellers
Of those 10 bank tellers, perhaps 9 are also active in feminist movements
That gives us:
10 bank tellers
9 feminist bank tellers
The mathematical truth becomes clear: there are always at least as many "bank tellers" as there are "bank tellers who are also feminists"—because every feminist bank teller is already counted in the bank teller group!
This is what Kahneman and Tversky called the conjunction fallacy: incorrectly believing that a specific combination of events (A and B) is more probable than a single event (A) alone.
The Golden Rule to Remember
The key takeaway is:
Adding more to a description can never make an event more likely.
Why It’s Hard to Accept
Even after understanding the logic, something might still feel off. As the renowned scientist Stephen Jay Gould confessed after learning about this problem:
“A little homunculus in my head continues to jump up and down, shouting at me—‘but she can’t just be a bank teller; read the description.’”
The issue comes from confusing two different concepts:
Representativeness: How well something matches our expectations or stereotypes
Probability: The actual mathematical likelihood of an event
As Kahneman explains: "The most coherent stories are not necessarily the most probable, but they are plausible, and the notions of coherence, plausibility, and probability are easily confused."
One More Example
Now that you know this, you will realise the conjunction fallacy being committed over and over again. Here is another example, also from Kahneman’s book:
Consider the following two scenarios:
A massive flood somewhere in North America next year, in which more than 1,000 people drown.
An earthquake in California sometime next year, causing a flood in which more than 1,000 people drown
(The answer should be obvious to you now!)
A Final Clarification
We have discussed several times in the past how new information should update our believes, and how this is done precisely using Bayes’ theorem.
You might wonder: "Doesn't the information about Linda's past make it more likely she's a feminist?"
Absolutely! Compared to a random woman, Linda is probably more likely to be involved in feminist activities. The description updates our probability assessment about that single attribute.
But that's not what the problem asks. Even though Linda is more likely than average to be a feminist, she's still more likely to be just a bank teller than a bank teller AND a feminist.
Remember this:
When it comes to probability: Less is more.
Next time you hear a complex, detailed prediction that sounds convincing because of its specificity, remember Linda. The more details added, the less likely it becomes—no matter how right it feels.
What other cognitive biases do you find fascinating? Let me know in the comments!
In case you missed it
The Hidden Psychology of Change: How We Value Gains and Losses
From Profit to Utility: Approaching Risk in Financial Decisions
How You Can Make Better Choices (Spoiler: Self-Interest Fails)
What do you want next?
I’ve seen this problem asked at job interviews!


Very interesting! Even knowing the explanation, a part of me still wants to say she is a feminist! Haha About the biasses, I've always been fascinated by the halo effect!